A Generic Self-Sustaining Process in Shear Flows
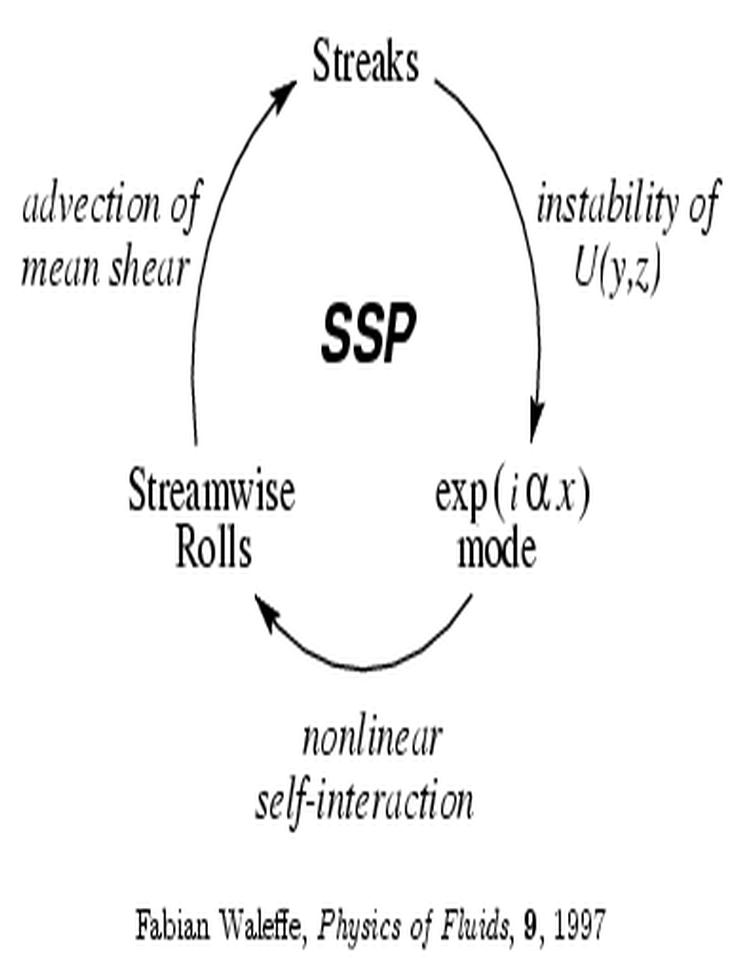
This nonlinear, three-dimensional Self-Sustaining Process
appears to be a generic mechanism in shear flows.
The mechanism has three main elements as depicted in the figure
above:
- Streamwise rolls sustain "streaks" (i.e. spanwise (z) modulation of the streamwise velocity),
by redistributing the mean momentum in cross-planes,
- the streaks suffer a wake-like instability due to the
spanwise inflections that leads to the onset of a streamwise ondulation,
- the nonlinear self-interaction of that streamwise ondulation directly
regenerates the streamwise rolls.
This process leads to self-sustained 3D traveling waves that
consists of wavy streaks flanked by staggered, counter-rotating,
quasi-streamwise vortices in both
plane Poiseuille flow
and
plane Couette flow with no-slip as well as free-slip
boundary conditions. This characteristic structure of the traveling waves
is very similar to the coherent structures revealed by pattern eduction
studies of turbulent
flows, hence we refer to the traveling wave solutions of the
Navier-Stokes equations as
Exact Coherent Structures .
The SSP also leads to time-periodic solutions as first revealed by
Waleffe and Kim (Turbulent Shear Flows conference, Munich 1991; see below),
Hamilton, Kim and Waleffe, Journal of Fluid Mechanics 287 ,
317-348 (March 1995) and more accurately by
Kawahara and Kida, Journal of Fluid Mechanics 449 , 291-300 (2001).
These authors improved on our earlier work by iterating toward an
"exact" (numerically speaking)
unstable periodic solution of the Navier-Stokes equations that is near the
flow we had obtained by successive "squeezing" of a turbulent flow, i.e.
tracking a self-sustained turbulent flow to smaller and smaller domains,
and thus Reynolds numbers. Our motivation was to reveal the importance and
relevance of the SSP.
(Contrary to what is often written, the SSP was not
discovered in Hamilton, Kim and Waleffe. That work was in fact aimed at verifying
the SSP proposed earlier
[W90]
and demonstrate that it was the key process sustaining shear turbulence.)
Squeezing the turbulent flow reduces the phase space
available to the flow and eliminates some of the "non-essential" instabilities
to reveal the basic process sustaining shear turbulence. That squeezing
technique was an adaptation of the "minimal channel idea" due to Jimenez and Moin
(JFM 1991). We focused on plane Couette flow because this flow is known
to be linearly stable and our understanding of the SSP was that the process
would operate within a single shear layer, so we expected it to occupy
the full channel at low Reynolds number in plane Couette flow.
In Poiseuille, we expected two copies of the process occuring in both half
of the channel.
The SSP approach has been used by
Faist and Eckhardt, PRL 91, 224502 (2003)
and Wedin and Kerswell, JFM 508:333-371 (2004)
to discover analogous traveling wave solutions in pipe flow.
Hof, van Doorne, Nieuwstadt and Westerweel, at
Delft University, were
able to make direct observations of these traveling waves in their
pipe flow experiments, see
Science 305, 1594-1598, Sept 10, 2004 .
- Recent publications
-
Homotopy of exact coherent structures in plane shear flows by
Fabian Waleffe, Physics of Fluids, 15, pp. 1517-1534 (June 2003).
- Exact coherent structures and their instabilities: Toward a dynamical-system theory of shear turbulence,
pp. 115-128
in Proceedings of the International Symposium
on ``Dynamics and Statistics of Coherent Structures in Turbulence:
Roles of Elementary Vortices'', Shigeo Kida, Editor,
National Center of Sciences, Tokyo, Japan, October 21-23, 2002
-
Exact Coherent Structures in Channel Flow
by Fabian Waleffe,
J. Fluid Mech., Vol. 435, pp. 93-102 (May 2001)
-
Three-Dimensional Coherent States in Plane Shear Flows
by Fabian Waleffe,
Phys. Rev. Lett., Vol. 81, Number 19, pp. 4140-4143 (9 Nov 1998)
- How Streamwise Rolls and Streaks Self-Sustain in
a Shear Flow: Part 2 by Fabian Waleffe and John Kim, AIAA paper 98-2997 (Albuquerque, June 1998).
-
On a self-sustaining process in shear flows by Fabian Waleffe,
Phys. Fluids, Vol. 9, pp. 883-900 (April 1997)
- How Streamwise Rolls and Streaks Self-Sustain in
a Shear Flow by Fabian Waleffe and John Kim in Self-Sustaining Mechanisms of Wall Turbulence
(R. Panton, Ed.), pp. 309-332, Computational Mechanics Publications, Southampton, UK
and Boston, USA, 1997.
-
Transition in shear flows: Nonlinear normality versus non-normal linearity by Fabian Waleffe.
Physics of Fluids 7, pp. 3060-3066.
[ps.gz]
-
Hydrodynamic Stability and Turbulence: Beyond transients to a self-sustaining process
by Fabian Waleffe.
Studies in Applied Mathematics, 95, pp. 319-343 (1995).
[ps.gz]
-
Regeneration mechanisms of
near-wall turbulence structures, by
James Hamilton, John Kim and Fabian Waleffe,
Journal of Fluid Mechanics, 287,
pp. 317-348 (March 1995) .
-
On the Origin of Streaks in Turbulent Shear Flows
by Fabian Waleffe, John Kim and James Hamilton.
Eds. F. Durst et al., in "Turbulent Shear Flows 8: selected papers from the
Eighth International Symposium on Turbulent Shear Flows, Munich, Germany, Sept. 9-11, 1991."
Springer-Verlag, Berlin, 1993.
[ps.gz]
- Proposal for a Self-Sustaining Mechanism in
Shear Flows by Fabian Waleffe, Center for Turbulence Research,
Stanford University/NASA Ames, Spring 1990. Unpublished.

