![jeanluc@[domainname],
where [domainname] is math.wisc.edu](Icons/jlt_email_wisc.gif){kind=icon}
| Lecture Room: | INGRAHAM B10 |
| Lecture Time: | MWF 1:20-2:10 |
| Lecturer: | Jean-Luc Thiffeault |
| Office: | 503 Van Vleck |
| Phone: | (608)263-4089 |
| Email: | |
| Office Hours: | 2:30–3:30 MW, or by appointment. |
| grade | # of students |
| A | 27 |
| AB | 20 |
| B | 36 |
| BC | 15 |
| C | 27 |
| D | 10 |
| F | 17 |
Class average: 2.55 (roughly BC)
Key: C = Thomas's Calculus, P = Precalculus
| lecture | date | sections | topic | homework |
| 1 | 01/21 | C3 | Review of derivatives | C p. 235: 1, 4, 5, 6, 10, 11, 13, 14, 25, 26, 36, 37, 40, 42, 46, 47, 63, 64, 65, 70, 73, 77, 108, 110 |
| 2 | 01/23 | C4.1 | Extreme values | C p. 252: 17–21, 28, 29, 35, 39, 40, 45, 48, 53 |
| 3 | 01/26 | C4.2 | Optimization; Mean value theorem | C p. 253: 55, 57, 60, 63, 66, 67; p. 260: 2–7, 23, 24, 26, 27, 31, 33, 34 |
| 4 | 01/28 | C4.3 | Monotonicity; First derivative test | C p. 266: 9–12, 10, 20, 24, 28, 30, 31 |
| 5 | 01/30 | C4.4 | Concavity; Second derivative test; Curve sketching | C p. 275: 9–13, 20, 23, 34, 35, 37, 39 |
| 6 | 02/02 | C4.4–4.5 | Curve sketching; More optimization | C p. 285: 2, 3, 4, 7, 14, 19 |
| 7 | 02/04 | C4.6 | L'Hopital's rule | C p. 298: 7, 8, 12, 15, 19, 23, 25, 28, 29 |
| 8 | 02/06 | C4.8;C5.1;C5.2 | Antiderivatives; Sigma notation | C p. 314: 1–6, 13–15, 17–22, 29–34, 49–52, 68, 69, 77, 78, 81, 94 |
| 9 | 02/09 | C5.2;C5.3 | Estimating area with finite sums; Definite integral | C p. 342: 1, 2, 5, 6, 7, 10, 17, 18; p. 352: 9, 10, 12, 14 |
| 10 | 02/11 | C5.3 | Area under a curve | C p. 353: 15, 19, 20, 27, 29, 30, 32, 37, 49, 50, 55, 56 |
| 11 | 02/13 | CA.1 | Mathematical induction | C p. AP-3: 2, 5, 9, 10 (in Appendix) |
| 12 | 02/16 | C5.4 | The fundamental theorem of calculus | C p. 365: 2, 3, 5, 6, 9, 14, 17, 19, 25, 28, 29, 30, 32, 33, 35 |
| 13 | 02/18 | C5.4;C5.5 | Area revisited; Substitution rule | C p. 365: 37, 38, 40, 41, 44, 47, 48, 51; p. 374: 1, 2, 4, 7, 11, 12, 14, 17, 24, 29, 41, 43, 44, 46, 48, 50, 54, 55, 56 |
| 14 | 02/20 | C5.6 | Substitution in definite integrals | C p. 383: 1, 3, 6, 8, 13, 14, 22, 26, 28, 32, 34, 39, 46, 50, 55, 56, 60 |
| 15 | 02/23 | C5.6; C6.1 | Area between curves; Volumes by slicing | C p. 405: 1, 3, 6, 9, 12, 15, 16, 25, 29. (see lecture 14 homework as well) |
| 16 | 02/25 | – | Midterm 1 | |
| 17 | 02/27 | C6.1; C6.2 | Washer method; Volume by shells | C p. 407: 31, 32, 36, 41, 44. p. 414: 2, 3, 8, 14, 22 |
| 18 | 03/02 | C6.1; C6.2 | More volume examples | |
| 19 | 03/04 | C6.4 | Moments and centers of mass | C p. 434: 3, 6, 8, 10, 12 (35, 36 removed) |
| 20 | 03/06 | P3-6; C7.1 | Inverse functions and their derivatives | C p. 473: 14, 16, 18, 19, 20, 21, 23, 27, 28, 30, 31, 33, 36 |
| 21 | 03/09 | C7.2 | Natural logarithms | C p. 484: 2, 3, 4, 5, 6, 10, 17, 18, 21, 24, 26, 28, 35 |
| 22 | 03/11 | C7.2 | Natural logarithms (cont'd); Logarithmic differentiation | C p. 484: 37, 38, 44, 49, 52, 54, 55, 60, 64, 68 |
| 23 | 03/13 | – | Exam tips; Example problems | |
| 24 | 03/23 | C7.3; C7.4 | Exponential function; General exponential and logarithm | C p. 493: 1, 2, 4, 7, 10, 15, 16, 20, 21, 23, 26, 35, 41, 48, 49, 54, 56, 63, 64 |
| 25 | 03/25 | C7.4; C7.5 | General exp. and log. (cont'd); Exponential growth | C p. 500: 1, 3, 4, 6, 10, 14, 15, 16, 30, 32, 39, 46, 50, 54, 60, 65, 69; C p. 508: 1, 3, 8, 12 (except d), 19 |
| 26 | 03/27 | C7.7 | Inverse trig functions | |
| 27 | 03/30 | C7.7 | Derivatives of inverse trig functions | C p. 530: 2, 6, 7, 8, 13, 14, 17, 23, 27, 29, 33, 45, 41, 46, 49, 50, 58, 62, 72, 75, 84, 88, 98, 99, 100, 104, 106, 109, 110, 111, 116 |
| 28 | 04/01 | – | Midterm 2 | |
| 29 | 04/03 | C7.8 | Hyperbolic functions | C p. 542: 5, 6, 10, 11, 14, 15, 16, 18, 19, 22, 23,24 |
| 30 | 04/06 | P8.1–8.2 | Law of sines and law of cosines | P p. 686: 1, 2, 6, 10, 13, 16, 18, 19, 23, 26, 29, 38. P p. 695: 1, 2, 6, 10, 12, 13, 14, 20, 22, 26, 27, 28, 30, 34, 37, 42 |
| 31 | 04/08 | C7.8 | Hyperbolic functions (cont'd) | C p. 543: 25, 26, 30, 33, 37, 41, 43, 46, 49, 51, 54, 57, 59. Also: find the error in the formula for inverse cosh in the table at the bottom of page 543. |
| 32 | 04/10 | C7.8 | The catenary; Special relativity | |
| 33 | 04/13 | P8.3 | Vectors | P p. 710: 1, 2, 4, 9, 12, 14, 15, 16, 18, 19, 22, 26, 29, 32, 33, 35, 38, 40, 41, 43, 46, 48, 49–56, 65, 71 |
| 34 | 04/15 | P1.4 | Complex numbers: introduction | P p. 126: 1–8, 13, 14, 15, 20, 24, 25, 27, 33, 35, 38, 39, 43, 46, 52, 53, 60, 63, 67, 76, 80, 81, 82 |
| 35 | 04/17 | P4.3 | Complex numbers: polynomials | P p. 410: 1, 3, 5, 6, 10, 11, 13, 16, 23, 25, 26, 27, 30, 32 |
| 36 | 04/20 | P8.5 | The complex plane | P p. 740: 3, 6, 7, 8, 9, 11, 12, 15, 17, 18, 19, 20, 22 |
| 37 | 04/22 | P8.5 | De Moivre's theorem | P p. 741: 23, 25, 26, 27, 30, 31, 34, 35, 37, 38, 40, 41, 42, 45, 46, 48, 50, 52 |
| 38 | 04/24 | P8.5 | Complex definition of sine and cosine | P p. 741: 63, 64, 67, 71, 72 |
| 39 | 04/27 | C8.3 | Partial fractions decomposition | C p. 579: 1, 2, 4, 6, 7, 10, 12, 13, 16, 18, 19, 20 |
| 40 | 04/28 | C8.3 | Partial fractions decomposition (cont'd) | C p. 579: 21, 23, 24, 25, 28, 29, 32, 35, 38 |
| 41 | 05/01 | C8.2 | Integration by parts | C p. 568: 1, 3, 6, 9, 12, 17, 20, 21, 23 (see example from class on 5/04 for the last two) |
| 42 | 05/04 | Review | C p. 318: 2, 3, 7, 12, 53, 54, 58, 61, 65, 69, 72, 91, 92; p. 389: 39, 40, 45, 47, 48, 56, 71, 72; p. 461: 2, 3, 7, 8, 9, 12, 13, 15; p. 510: 19, 20, 26; p. 547: 1, 4, 7, 12, 14, 16, 19, 24, 25, 27, 28, 30, 31, 37, 40, 43, 52, 53, 59, 66, 70, 71, 74, 77, 78, 79, 81, 84, 85, 92, 93, 94, 96. | |
| 43 | 05/06 | Review | C p. AP-21: 2, 11, 12, 13, 14 (Argand diagram means the same as complex plane), 19, 20; P p. 740: 16, 18, 23, 26, 27, 28, 46, 50 (note: no review exercises on partial fractions/integration by parts will be assigned, since this was the last thing covered. However, they are included in the final.) | |
| 44 | 05/08 | Review | See exercices from 05/04 and 05/06. Note that the exercises are not exhaustive: they might not cover absolutely everything that could be on the final. Review the homeworks as well. Updated: curve sketching exercises: C p. 320: 23, 23, 26, 31, 34, 45, 49 |
The official prerequisite is Math 171.
See the official syllabus.
The textbooks for the class are
Each week I will assign homework from the textbooks and post it here (above). Each following Tuesday (starting in the second week of class), your TA will give a 20 min quiz during your discussion section, consisting of a few questions from or related to the previous few homeworks. This will make up part of your grade, as described below. The TA for your section might decide to collect some homework or have some additional methods of assessment, at their discretion.
Notes, textbooks, or calculators will not be allowed in the quizzes. At least one of your lowest quiz scores will be discarded, and there will be no make-up quizzes.
Even if it is not collected, you should do all of the homework if you want a chance to do well in the class.
| Name | Office | Phone | |||
| Jie Ling | 718 Van Vleck | 2-0079 | ling | @ | math.wisc.edu |
| Sarah Matz | 418 Van Vleck | 2-0011 | matz | @ | math.wisc.edu |
| Dan Rosendorf | 422 Van Vleck | 3-2410 | rosendor | @ | math.wisc.edu |
| Michael Woodbury | 422 Van Vleck | 3-2410 | woodbury | @ | math.wisc.edu |
| Number | Time | Days | Room | TA |
| 322 | 8:50 | TR | B321 VAN VLECK | Woodbury |
| 323 | 8:50 | TR | 215 INGRAHAM | Ling |
| 325 | 11:00 | TR | B235 VAN VLECK | Woodbury |
| 328 | 13:20 | TR | B135 VAN VLECK | Matz |
| 329 | 14:25 | TR | B203 VAN VLECK | Matz |
| 334 | 9:55 | TR | 215 INGRAHAM | Ling |
| 335 | 11:00 | TR | B131 VAN VLECK | Rosendorf |
| 337 | 12:05 | TR | 123 INGRAHAM | Rosendorf |
There will be two midterm exams. Each of the two midterm exams is worth 20 percent, for a total of 40 percent of the final grade. The final exam will count for 40 percent. The remaining 20 percent is a Discussion Section grade allocated by your TA who will base it on homework, quizzes, participation, attendance, and effort. (The Discussion Section grade will be adjusted to account for variations among the TAs.)
| Midterm Exam I | 20% | ( | |
| Midterm Exam II | 20% | ( | |
| Final Exam | 40% | (Monday May 11, 2009 at 10:05 A.M.) | |
| Discussion section grade | 20% |
Calculators, notes, and textbooks are not allowed in exams or quizzes. The intelligent use of calculators outside of exam rooms is however encouraged.
There are many ways to get help with math. In addition, following these guidelines is a recipe for (but not a guarantee of) success:
| # of scores | 155 |
| mean score | 61.46 |
| standard deviation | 19.2 |
| median score | 61.0 |
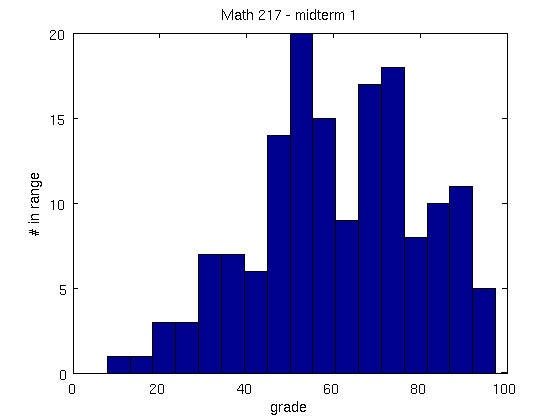
Midterm 1 solutions (courtesy of Sarah)
| # of scores | 153 |
| mean score | 75.08 |
| standard deviation | 18.46 |
| median score | 80 |
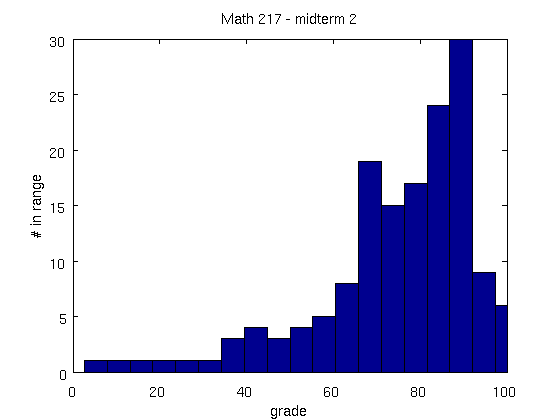
Midterm 2 solutions (courtesy of Jie)
|
|
Last modified: 19:39 1 April 2021 |
Background: Coarsening of a binary fluid (Simulations by Lennon Ó Náraigh) |
Follow @jeanluc_t |
|