Jean-Luc Thiffeault

Bubbles and Filaments: On the Advective Cahn–Hilliard Equation

Lennon Ó Náraigh (with Jean-Luc Thiffeault)

At the moment I am studying the advective Cahn–Hilliard equation. This is an equation which describes what happens when you stir two immiscible fluids like oil and water. Such a combination is called a binary fluid. Without stirring, the equilibrium state of the system is collection of bubbles of oil and water. However, by stirring hard enough, I have found that it is possible to obtain a new equilibrium state in which the oil and the water are well mixed.

The model without flow was introduced by Cahn and Hilliard in 1957 [1] to describe how a molten alloy like iron and nickel separates into its component parts. The domain or bubble solution is to be thought of in terms of a free energy functional:

∫ [ ] n 1( 2 )2 γ- 2 F [c] = Ω d x 4 c - 1 + 2 ∣∇c ∣ ,

where c is the concentration: c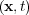 (x,t) = 1 for pure iron and c (x,t) = -1 for pure nickel. γ is a parameter which gives the thickness of the layer separating the bubbles of iron and nickel. The concentration evolves in time by what is called flow by the Laplacian of the gradient:

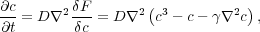
( ) ∂c = D ∇2δF- = D∇2 c3 - c- γ∇2c , ∂t δc

where D is a constant governing the rate of separation of the alloy into its components.

I add a flow to this equation to try to rehomogenize the concentration. I choose a special flow that is chaotic, so that nearby fluid particles separate in exponentially fast as the stirring takes place. The rate of separation is called the Lyapunov exponent and this depends on how vigorously you stir the fluid. The picture now is of a molten alloy, or a polymer blend, or oil and water - in any state you like, being stirred in an optimal way. The segregating tendencies of such a mixture are now either stopped, giving bubbles of a particular size, or completely overcome, giving a well-mixed state.

In symbols the equation for the stirred case is this:

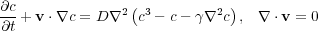
∂c+ v ⋅∇c = D∇2 (c3 - c- γ∇2c ), ∇ ⋅v = 0 ∂t

and in pictures the results given above are the following:

Figure 1:

The concentration field after t = 30,000 timesteps in dynamical equilibrium, for various stirring amplitudes α.

As with previous studies of the no-flow situation [2, 3], I find that the bubbles grow indefinitely as t^1∕3, where t is time. The exponent is called the Lifshitz-Slyozov exponent, after the people who discovered this law [4]. However, with the chaotic flow, there are two regimes:

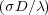For small stirring amplitudes α there are bubbles with jagged edges. These have a typical radius that is constant in time and equal to ^1∕3. The 1∕3 exponent that appears here is not a coincidence. σ is the surface tension and λ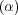 is the Lyapunov exponent mentioned before.
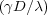For larger stirring amplitudes the bubbles are no longer prominent (although they are still there). They are replaced by long wispy regions called filaments. The width of the filament depends on the Lyapunov exponent in a complicated way. By studying a simplified one-dimensional model, I have found that this width can never be greater than ^1∕4, which is called the hyperdiffusive limit.

Some more relevant papers on the subject are those by Berti et al [5], Berthier et al [6]. A review of the subject from a theoretical point of view is given by Bray [7].

References

[1] J.W. Cahn and J.E. Hilliard. Free energy of a nonuniform system. I. Interfacial energy. J. Chem. Phys, 28:258–267, 1957.

[2] J. Zhu, L.Q. Shen, J. Shen, and V. Tikare. Coarsening kinetics from a variable mobility Cahn–Hilliard equation: Application of a semi-implicit Fourier spectral method. Phys. Rev. E, 60:3564–3572, 1999.

[3] A.J. Bray. and C.L. Emmott. Lifshitz-Slyozov scaling for late-stage coarsening with an order-parameter dependent mobility. Phys. Rev. B, 52:R685, 1995. They prove the more general result that for 1D ∝ 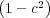(1 - c2) ^α, the typical domain size R_d (t) grows in time as t^1∕.

[4] I.M. Lifshitz and V.V. Slyozov. The kinetics of precipitation from supersaturated solid solutions. J. Chem. Phys. Solids, 19:35–50, 1961.

[5] S. Berti, G. Boffetta, M. Cencini, and A. Vulpiani. Phase separation in a chaotic flow. Phys. Rev. Lett., 95:224051, 2005.

[6] L. Berthier, J.L. Barrat, and J. Kurchan. Phase separation in a chaotic flow. Phys. Rev. Lett., 86:2014–2017, 2001.

[7] A.J. Bray. Theory of phase-ordering kinetics. Adv. Phys., 43:357–459, 1994.