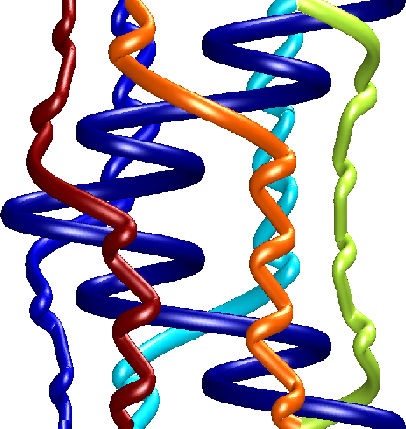
The braid formed by the world lines of a rod and several periodic islands in a mixing device.
In recent years, starting with the work of Boyland et al. [2, 3], methods from topology and braid theory have
been applied to the analysis of mixing in flows with great success. Consider a mixing device consisting of rods moving
in a two-dimensional viscous fluid. If the position of the rods is plotted in a three-dimensional graph, with time the
vertical axis, one obtains a “spaghetti plot” of the world lines of the rods, similar to the plot shown here. Because the
rods are material objects, the world lines cannot intersect each other. The resulting graph thus describes
a braid, in the mathematical sense of a bundle of strands that are not allowed to cross each other.

The braid formed by the world lines of a rod and several periodic islands in
a mixing device.
The analysis of this braid yields important information about the mixing properties of the flow. For instance, it can be shown that if the braid possesses a positive topological entropy, then there must exist a region in the surrounding fluid that exhibits chaotic trajectories. It is well-known [1] that chaotic trajectories are very good for mixing, especially in very viscous flows where turbulence is nonexistent. The presence of chaos is a consequence of the topology of the rod motion, and is guaranteed no matter which dynamical equations the fluid obeys—hence the name topological chaos.
The reasoning for the presence of chaos involves material lines in the fluid, which are lines that are assumed to move with the fluid, in the manner that a nondiffusing blob of dye would. A material line is dragged and stretched by the fluid motion, but it cannot cross the physical rods. Hence, the material lines inherit the complexity of the rod motion because it “snags” onto the rods. If the braid formed by the rods has positive topological entropy, then material lines must grow exponentially, ensuring chaos.
But physical rods are not necessary for topological chaos. Any particle orbit is an obstacle to material lines [5]. Periodic orbits have been particularly useful in characterising topological chaos, and the most appealing periodic orbits for this purpose are regular islands, also known as elliptic regions. The graph on this page shows the motion of a single rod as well as regular islands created in the viscous flow by the motion of the rod. Topologically speaking, there is no difference between the rod and the islands, and the braid shown here has positive topological entropy [4]. This opens up the intriguing possibility of optimising mixing devices by altering the braid formed by the motion of rods and islands.
[1] H. Aref, Stirring by chaotic advection, J. Fluid Mech., 143 (1984), pp. 1–21.
[2] P. L. Boyland, H. Aref, and M. A. Stremler, Topological fluid mechanics of stirring, J. Fluid Mech., 403 (2000), pp. 277–304.
[3] P. L. Boyland, M. A. Stremler, and H. Aref, Topological fluid mechanics of point vortex motions, Physica D, 175 (2003), pp. 69–95.
[4] E. Gouillart, M. D. Finn, and J.-L. Thiffeault, Topological mixing with ghost rods, Phys. Rev. E, 73 (2006), p. 036311.
[5] J.-L. Thiffeault, Measuring topological chaos, Phys. Rev. Lett., 94 (2005), p. 084502.