|
Research |
|
My research focuses on differential geometry and geometric analysis (partially supported by NSF grant DMS-2304818), including Ricci curvature, Calabi-Yau spaces, sectional curvature and conformal invariants. My primary research interests are as follows:
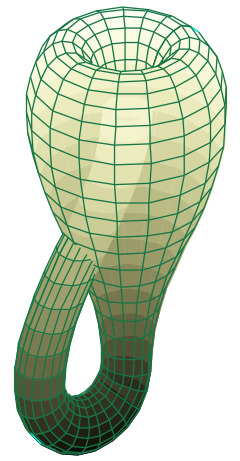
Collapsing geometry of special Einstein metrics
We summarize some recent results on collapsing Calabi-Yau manifolds, i.e., hyperkähler manifolds, in real dimension 4; see my joint paper [SZ21] with Song Sun.
Let us take the K3 manifold $\mathcal{K}$ for an example.
Let $g_j$ be a sequence of Calabi-Yau metrics on $\mathcal{K}$ which are collapsing to some compact limit metric space $(X_{\infty}^d, d_{\infty})$. We proved in [SZ21] that the Hausdorff dimension $d\equiv \dim_{\mathcal{H}}(\mathcal{K})$ is always an integer. Namely, $d\in\{0,1,2,3\}$. We also obtained the following structure results.
($d=3$) a flat orbifold $\mathbb{T}^3/\mathbb{Z}_2$;
($d=2$) a topological sphere $S^2$ equipped with a singular special Kähler metric;
($d=1$) a closed interval.
It is worth pointing out that the limit space $(S^2,d_{\infty})$, as a singular special Kähler manifold, is an Alexandrov space with nonnegative curvature (also RCD(0,2) space).
($d=3$) $C(W) \equiv \mathbb{R}^3$ or $\mathbb{R}^3/\mathbb{Z}_2$,
($d=2$) $C(W) \equiv C(S_{2\pi\beta}^1)$ with $\beta\in\{\frac{1}{2}, \frac{1}{3}, \frac{2}{3}, \frac{1}{4}, \frac{3}{4}, \frac{1}{6}, \frac{5}{6}, 1\}$,
($d=1$) $C(W)\equiv [0,\infty)$.
(Dimension monotonocity) any bubble limit $(Z_{\infty}^k, d_{Z}, z_{\infty})$ has integer Hausdorff dimension $k\geq d$.
(Canonical bubbles) there exists a unique scale $\lambda_j\to\infty$ that gives a bubble limit
$(Z_{\infty}^4, d_{Z}, z_{\infty})$ and satisfies the following.
The $\epsilon$-regularity theorem of collapsing Einstein spaces
Joint with Aaron Naber, we proved in [NZ16] the first $\epsilon$-regularity theorems for higher dimensional collapsing Einstein spaces. It relates
a priori curvature estimates with Gromov-Hausdorff behavior, which is entirely new in the collapsed context.
Mainly it states that, if a ball $B_2(p)$ is Gromov-Hausdorff close to a $k$-dimensional metric space,
then the group generated by short loops
\begin{equation}\Gamma_{\epsilon}(p)=\text{Image}[\pi_1(B_{\epsilon}(p))\longrightarrow\pi_1(B_2(p))]\end{equation} is virtually nilpotent with
$\text{Nilrank}(\Gamma_{\epsilon}(p))\leq n-k$. More importantly, $\text{Nilrank}(\Gamma_{\epsilon}(p))= n-k$ implies gives uniform curvature estimates in $B_1(p)$.
In particular, if $B_2(p)$
is collapsing to a ball in $\mathbb{R}^k$, then an $\epsilon$-regularity holds iff $\text{Nilrank}(\Gamma_{\epsilon}(p))= n-k$.
The crucially new nature of this regularity result is that uniform curvature estimates can be reduced to simple topological detections which can be conveniently applied in many different contexts.
Geometric structures of collapsed Ricci limits:
A gravitational instanton $(X^4, g)$, by definition, is a complete hyperkähler manifold with quadratic integrable curvature. Notice that under the Einstein condition, the $L^2$-energy finiteness is equivalent to the topological finiteness. As described above, gravitational instantons naturally
appear as bubble limits of degenerating Calabi-Yau metrics in complex dimension $2$.
Our recent work [SZ21]
addresses a folklore conjecture in this field which gives a complete classification for the asymptotic structures of gravitational instantons. That is, any gravitational instanton $(X^4, g)$ has a unique asymptotic cone $Y^d$ which is a flat metric cone. Moreover, $(X^4,g)$
must have one of the following asymptotic models: ALE, ALF, ALG, ALH, ALG$^*$, and ALH$^*$.
An important application of the above classification theorem involves the compactification of gravitational instantons.
Given a fixed gravitational instanton, there are extensively studies on the compactification in the complex geometric sense.
Based on the above classification result proved in [SZ21] as well as the known compactification results, we can conclude that all gravitational instantons can be compactified, which also confirms Yau's compactification conjecture in the case of gravitational instanton.
Nilpotent structures on K3 surfaces:
In [HSVZ22] (joint with Hans-Joachim Hein, Song Sun and Jeff Viaclovsky), we exhibit a family of Ricci-flat Kähler metrics on K3 surfaces which collapse to a closed interval $[0,1]$ with Tian-Yau space $X_{TY}^4$ and Taub-NUT space $\mathbb{C}_{TN}^2$ occurring as bubbles.
There is a singular nilpotent fibration over the interval such that nilpotent fibers change topologies when crossing the singularities.
Degenerations and metric geometry of collapsed Calabi-Yau spaces:
In the joint work [SZ19] with Song Sun,
we make progress on understanding the collapsing behavior of Calabi-Yau metrics on a degenerating family of polarized Calabi-Yau manifolds.
An especially
intriguing phenomenon is that Calabi-Yau metrics may collapse with highly non-algebraic features.
In the case of a family of smooth Calabi-Yau hypersurfaces $(X_t^{2n},g_t)\subset \mathbb{P}^{n+1}$ degenerating into the transversal union of two smooth Fano hypersurfaces in a generic way:
$$f_1(x)\cdot f_2(x) + t\cdot f(x) = 0, \quad t\to 0,\quad x\in \mathbb{P}^{n+1}.$$
In this setting, we are able to precisely characterize the delicate Riemannian and complex geometry of $g_t$:
Type II degeneration of quartic K3 surfaces
higher dimensional degenerations of quintic Calabi-Yau threefolds in $\mathbb{P}^4$.
Remark:
The degeneration theory developed in [HSVZ22] and [SZ19] is related to various duality phenomena and domain walls in superstring theory. Such relations have been recently
established by in
[CH19a, CH19b]),
New bubbling behaviors of collapsing Calabi-Yau manifolds:
New bubbling phenomena have been discovered in our recent studies.
For instance,
in [HSVZ22],
the Tian-Yau spaces, for the first time, were realized as bubbles of collapsing K3 surfaces.
With technically much more involved, this type of collapsing scenario was extended to higher dimensions in [SZ19]. In another direction, together with Gao Chen and Jeff Viaclovsky, our work in [CVZ20] is to investigate the collapsing hyperkähler metrics on an elliptic K3 surface which are collapsing to a singular metric on $\mathbb{P}^1$.
We managed to understand the singularity behavior around each type of singular fibers (in Kodaira's classification). It is worth mentioning that
we obtained the first degeneration with complete ALG spaces (satisfying $\text{Vol}(B_R)\sim R^2$ and $|\text{Rm}|\sim R^{-2}$) occurring as bubble limits of collapsing K3 surfaces.
In the generic case of our construction, a deepest bubble is asymptotic to $\mathbb{T}^k$-bundle over an ALE space for $k\in\{0,1,2\}$, which may occur in the meanwhile.
Moduli space of K3 manifolds:
We have made progress in understanding the moduli space of K3 surfaces $\mathfrak{M}$. Using Satake's compactification, Odaka-Oshima ([OO18]) have identified the boundary $\partial \mathfrak{M}$ as having $6$ strata.
The degeneration
analysis established in our recent work help understand the structure of $\mathfrak{M}$ near the boundary strata.
For instance, Constructions in [CVZ20] give an open subset in $\mathfrak{M}$ which contains the 36-dimensional boundary stratum. As a comparison, the hyperkähler metrics obtained in [HSVZ22]
constitute an open set in $\mathfrak{M}$ containing a $2$-dimensional boundary stratum.
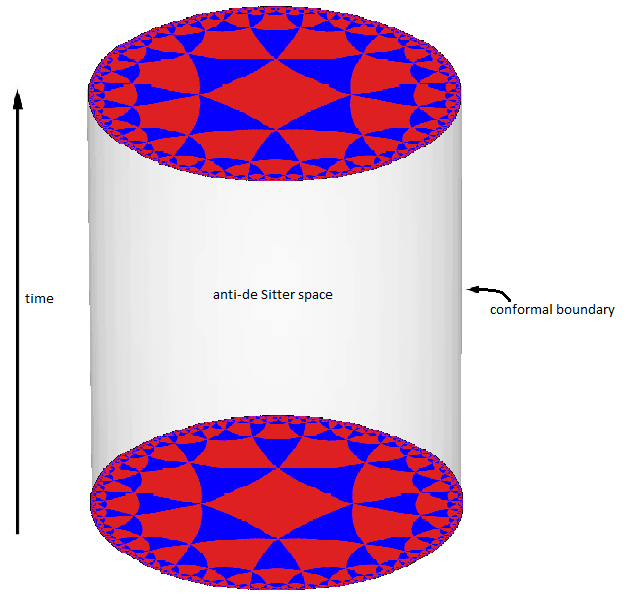For a Poincaré-Einstein space $(X^{n+1}, g)$
with a conformal infinity $(M^n,[h])$, a central topic is to explore their connections.
A way in understanding this is to implement nonlocal analysis with the Dirichlet-to-Neumann operators $P_{2\gamma}$ with a leading term $(-\Delta)^{\gamma}$
and associated curvatures $Q_{2\gamma}$,
which originates from geometric scattering theory and effectively unifies
conformal invariants of different orders.
For instance, scalar curvature and the Branson's Q curvature occur as $\gamma=1$ and $\gamma=2$ respectively.
In [Zhang16], we obtain
a sharp estimate for the complexity of Kleinian group structure
which obstructs the existence of metrics $Q_{2\gamma}\geq 0$ of a Poincé-Einstein space.
This result can be viewed as a nonlocal version of Schoen-Yau's fundamental result in the case $\gamma=1$ ([SY88]).
As applications, we also obtained topological rigidity and classification theorems for the manifolds with $Q_{2\gamma}\geq 0$.
Recently in [CZ19] (joint with Wenxiong Chen), we obtained several regularity and isometric rigidity theorems for the conformally flat metrics with constant curvature $Q_{2\gamma}$ as $\gamma=\frac{n}{2}$. This requires delicate regularity and geometric analysis
for a new type of nonliearity, which arises from the scattering behavior
in the limiting case.
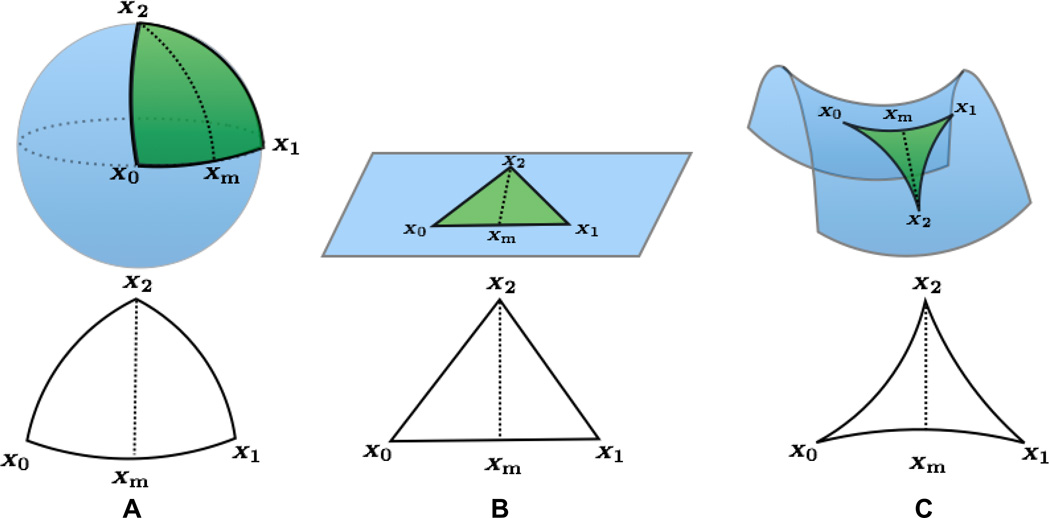
(A) Metric geometry of Ricci curvature and Einstein manifolds
(i) Gromov-Hausdorff collapsed limits:
The limit space $(X_{\infty}^d, d_{\infty})$ must be isometric to one of the following.(ii) Tangent cones:
The tangent cone at each singular point $q_{\infty}\in X_{\infty}^d$ is unique and must be a flat metric cone $(C(W),d_C)$:(iii) Bubble tree structure:
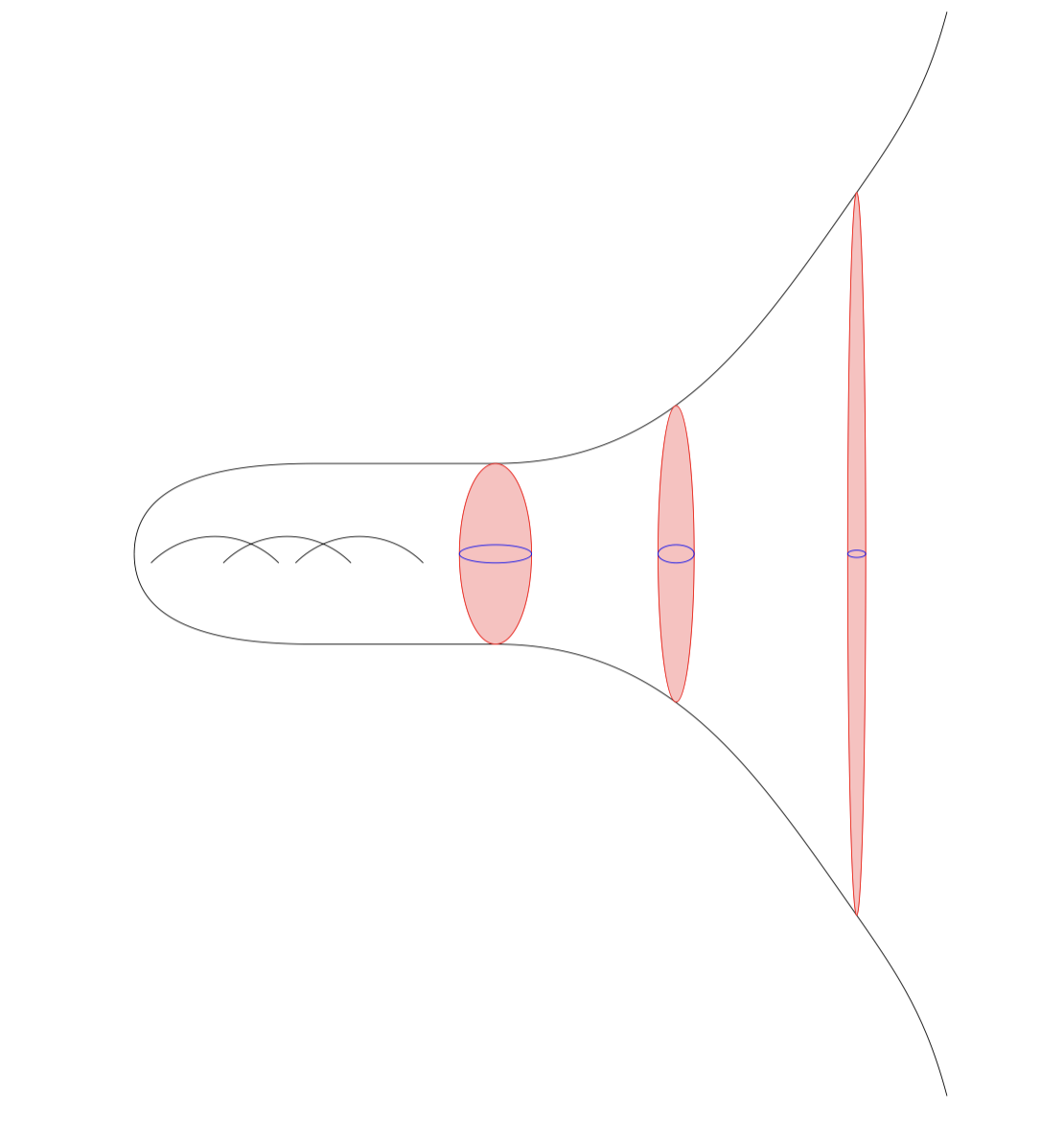
Analyzing bubbles is always a central theme in any kind of nonlinear analysis. For any converging sequence $(X_j, g_j, x_j)$, a bubble at $x_j$, by definition, is just a rescaled limit $(X, \lambda_j^2 g_j, x_j)\xrightarrow{GH}(Z_{\infty}, d_{Z_{\infty}}, z_{\infty})$ for some rescaling sequence $\lambda_j\to\infty$. In our context, let $(M_j^4,g_j,x_j)$ be a sequence of Calabi-Yau manifolds that satisfies $$\int_{B_2(x_j)}\|\text{Rm}_{g_j}\|^2 \leq \Lambda $$ and yields the convergence $(X_j, g_j, x_j) \xrightarrow{GH} (Y_{\infty}^d, d_{Y}, y_{\infty})$, where $d\equiv\dim_{\mathcal{H}}(Y_{\infty}^d)$. Then we have the following rather complete characterization of the bubble limits.- (a) $(Z_{\infty}^4, d_{Z}, z_{\infty})$ is a hyperkähler orbifold.
- (b) $\text{Vol}(B_r(z_{\infty}))$ grows at least $O(r^k)$ as $r\to\infty$.
- (c) Any scale $\lambda_j' \gg \lambda_j$ gives the Euclidean bubble limit $\mathbb{R}^4$ and any scale
$\lambda_j' \ll \lambda_j$ gives bubble limits with dimension strictly less than $4$.
(i) Nilpotent structures:
For a sequence of collapsing manifolds with uniformly bounded curvatures, if they collapse to a metric space $(X_{\infty}^k,d_{\infty})$ with maximal nilpotent rank $Nilrank(\Gamma_{\epsilon}(p))= n-k$, then the nilpotency can be strengthened as follows: $X_{\infty}^k$ is locally isometric to $Y^n/\mathcal{N}^k$ for some metric space $Y^n$ and nilpotent Lie group $\mathcal{N}^k\leq \text{Isom}(Y^n)$.(ii) Regularity:
In the above context, by [NZ16], the full measure regular set in $X_{\infty}^k$ is a smooth orbifold. In a special case, if $g_j$ are a hyperkähler sequence on a K3 manifold $\mathcal{K}$, then away from finite points, $g_j$ are close to the Gibbons-Hawking metric with nilpotent symmetries. More precise picture is characterized in [HSZ19] (joint with Shouhei Honda and Song Sun).(iii) Canonical affine structure:
It is shown in [HSZ19], we discovered canonical affine structures for hyperkähler limits. If hyperkähler manifolds $(\mathcal{K},g_j)$ are collapsing to $[0,1]$ with $\frac{dvol_{g_j}}{\text{Vol}_{g_j}(\mathcal{K})}\to \nu_{\infty}$, then there is a canonical affine coordinate system $\{z\}$ on $[0,1]$ so that the limit volume density $V_{\infty}(z)$ is piecewise linear with non-smoothing loci precisely corresponding to the singularities of $g_j$. This is analogous to large complex structure limits in mirror symmetry theory, where one expects integral affine structure with singularities (see [Gr12]).(iv) Limiting metric-measure geometry:
By [HSZ19], the hyperkähler limit $([0,1], dt^2, \nu_{\infty})$ is a $\text{RCD}(0,\frac{4}{3})$ metric-measure space with $\text{Ric}\geq 0$ and $\dim\leq \frac{4}{3}$, and the optimal dimension either $1$ or $\frac{4}{3}$. In fact, $\frac{4}{3}$-case is realized in [HSVZ22].(B) Geometry of gravitational instantons
A gravitational instanton $(X^4, g)$, by definition, is a complete hyperkähler manifold with quadratic integrable curvature. Notice that under the Einstein condition, the $L^2$-energy finiteness is equivalent to the topological finiteness. As described above, gravitational instantons naturally
appear as bubble limits of degenerating Calabi-Yau metrics in complex dimension $2$.
Our recent work [SZ21]
addresses a folklore conjecture in this field which gives a complete classification for the asymptotic structures of gravitational instantons. That is, any gravitational instanton $(X^4, g)$ has a unique asymptotic cone $Y^d$ which is a flat metric cone. Moreover, $(X^4,g)$
must have one of the following asymptotic models: ALE, ALF, ALG, ALH, ALG$^*$, and ALH$^*$.
An important application of the above classification theorem involves the compactification of gravitational instantons.
Given a fixed gravitational instanton, there are extensively studies on the compactification in the complex geometric sense.
Based on the above classification result proved in [SZ21] as well as the known compactification results, we can conclude that all gravitational instantons can be compactified, which also confirms Yau's compactification conjecture in the case of gravitational instanton.
(C) Collapsing and degenerations in complex geometry
(a) Let us renormalize $\text{Diam}_{g_t}(X_t)=1$. Then the limit space is $[0,1]$ and singularities occur only at rational points. In addition, it is an $\text{RCD}(0,\frac{2n}{n+1})$ space with optimal dimension $\frac{2n}{n+1}$.
(b) There is a singular fibration $\mathscr{F}_t:X_t \to [0,1]$ with graded collapsing fibers.(c) Bubble limits can be explicitly claissified. For example, different bubbles such as the Tian-Yau space and the product gravitational instanton $\mathbb{C}_{TN}^2\times \mathbb{C}^{n-2}$ appear in the collapsing sequence.
We also exhibit an effective way to produce both complete and incomplete Calabi-Yau metrics, which is of independent interest. Typical examples:(D) Poincaré-Einstein manifolds and conformal invariants
For a Poincaré-Einstein space $(X^{n+1}, g)$
with a conformal infinity $(M^n,[h])$, a central topic is to explore their connections.
A way in understanding this is to implement nonlocal analysis with the Dirichlet-to-Neumann operators $P_{2\gamma}$ with a leading term $(-\Delta)^{\gamma}$
and associated curvatures $Q_{2\gamma}$,
which originates from geometric scattering theory and effectively unifies
conformal invariants of different orders.
For instance, scalar curvature and the Branson's Q curvature occur as $\gamma=1$ and $\gamma=2$ respectively.
The following is my recent research in this direction: