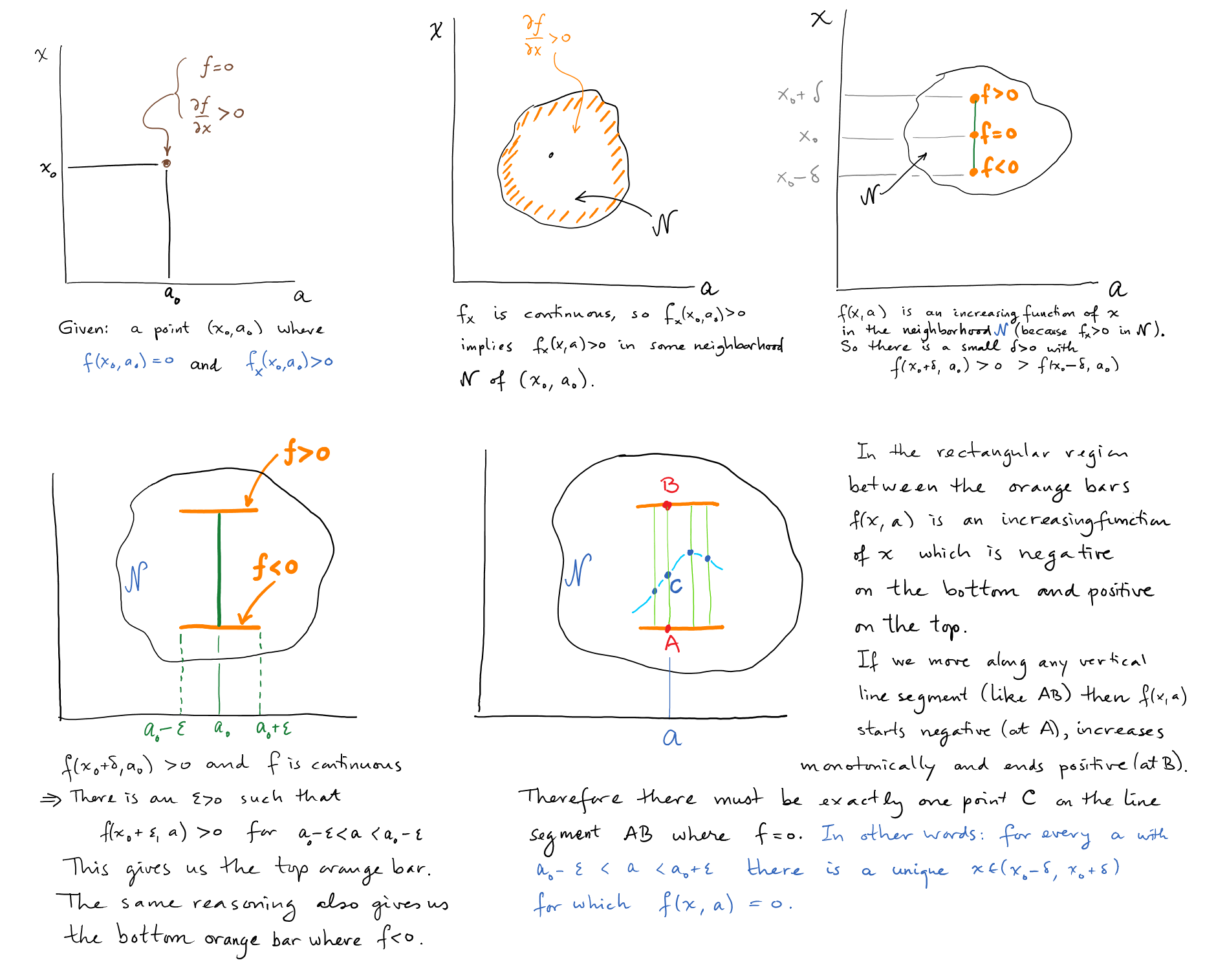
The Implicit Function Theorem.
Let $f(x, y)$ be a function defined on some plane domain with continuous
partial derivatives in that domain, and suppose that a point $(x_0, y_0)$ in
the zero set of $f$ is given.
-
If $\frac{\pd f}{\pd y}(x_0, y_0) \neq 0$ then there is a small
rectangle centered at $(x_0, y_0)$ such that within this rectangle the
zero set of $f$ is the graph of a differentiable function $y=\varphi(x)$.
-
If $\frac{\pd f}{\pd x} (x_0, y_0) \neq 0$ then there is a small
rectangle centered at $(x_0, y_0)$ such that within this rectangle the
zero set of $f$ is the graph of a differentiable function $x=\psi(y)$.

The Implicit Function Theorem. The zero set of a
function $f(x, y)$ does not have to be the graph of a function, but
if at some point ($A$) on the zero set we have $f_y\neq0$, then,
near that point $A$, the zero set is the graph of a function
$y=\varphi(x)$. If $f_x\neq0$ at some point ($B$), then near $B$ the zero
set is also the graph of a function, provided we let $x$ be a
function of $y$ : $x=\psi(y)$.